Fick’s Law is a principle of physics, and forms the basis and foundation of diffusion and more generally mass transfer. Diffusion is the action where molecules of dye in water spread out out from their source instead of staying concentrated where the source is, even if you don’t mix up the water at all. In layman’s terms I would define it like this: “molecules diffuse from areas of high consentration to areas of low concentration. They diffuse faster when the difference in concentration between the areas is larger, and the diffuse faster when the distance between the areas is smaller.”
A more accurate definition would be stated like this: “the diffusive flux is proportional to the difference in concentration, and inversely proportional to the distance.” First of all let’s look at the word ‘flux’, a very technical term. It’s basically a way of quantifying how much ‘stuff’ is moving at any particular point in space. It’s defined by the following:
\( \large{ \left[ \text{flux of stuff} \right] = \large{ \frac{\left[ \text{stuff} \right]}{\left[ \text{area} \right] \left[ \text{time} \right] } } } \)
So basically flux means the rate of flow of stuff per unit area. The ‘stuff’ is for whatever moving quantity you are interested in. For example in SI units molar flux would be in units of mol/(m2·s), heat flux (or more generally energy flux) would be J/(m2·s) which is equivalent to W/m2, and mass flux would be kg/(m2·s). A bit more esoterically in fluid dynamics there is momentum flux, information theory has entropy flux, electromagnetism uses electric flux and magnetic flux, and quantum mechanics even has probability flux!
Anyway, in the definition for Fick’s Law the word ‘proportional’ means a linear relationship: if the difference in concentration doubles, then the flux doubles. If the difference in concentration reduces to one half, then the flux reduces to one half as well. ‘Inversely proportional’ means the opposite: if the distance doubles, then the flux halves. If the distance halves, then the flux doubles.
For example, look at the following simple example:
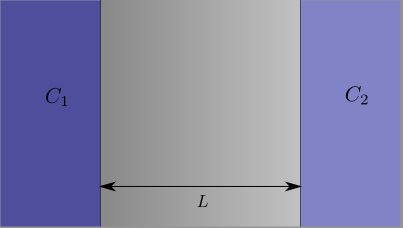
On the left we have some medium that contains substance \(A \) at concentration \(C_1 \), separated by a membrane of thickness \(L \) from another medium that contains substance \(A \) at some lower concentration \(C_2 \). Substance \(A \) then diffuses from the left through the membrane to the right.
From the definition of Fick’s Law we know that it’s the difference between the concentrations on the left and the right that’s important. We’ll define this as \(\Delta C = C_2 – C_1\).
So now if we define the flux of \(A \) through the membrane as \(N_A \), then we can say the following:
1. \(N_A \) is proportional to \(\Delta C\), which is equivalent to
\( N_A = \left[ \text{constant} \right] \times \Delta C \)
2. \(N_A \) is inversely proportional to \(L \), which is equivalent to
\( N_A = \left[ \text{constant} \right] \times \frac{ 1 }{ L } \)
3. We can then combine the two statements to say:
\( N_A = \left[ \text{constant} \right] \times \frac{ \Delta C }{ L } \)
Basically we’re just combining the two constants into a single conglomerate constant. We can do this because while we have stated it’s a constant, we haven’t stated what value it must be, so we can combine multiple constants and call it a single constant.
So what do we do for this constant? The above equation tells us that so long as the material of the membrane separating the two remains the same and that it is substance \(A \) that is diffusing through it remains the same, \(\Delta C \) and \(L \) can change to practically any value and the value of the constant will not not change. So it becomes a property of the material that is being diffused through.
We call this constant the diffusivity, and use the notation \(D_{AB} \), which denotes ‘diffusivity for substance \(A \) diffusing through substance \(B \)’.
So now we can write Fick’s Law as a general equation:
\( \huge{ N_{A} = – D_{AB} \frac{\Delta C_A}{ L } } \)
First of all, what’s with the negative sign? This basically tells us that the species \(A \) if flowing from areas of high concentration to low concentration, assuming that \(D_{AB} > 0 \) (which it is by definition). Otherwise we would have things spontaneously flowing from low concentration to high concentration, which violates the 2nd law of thermodynamics.
What are the units on \(D_{AB} \)? We know the units of everything else in the equation, so it’s fairly simple to work out. Units of the molar flux \(N_A \) is mol/(m2·s), concentration difference \(\Delta C \) is mol/m3, and distance \(L \) is of course simply m. So units for \(D_{AB} \) work out be m2/s.
Some typical values of \(D_{AB} \) are shown below:
\(\small{\begin{array}{ccc} \text{solute} \: A & \text{medium} \: B & D_{AB} \: [\text{m}^2 / \text{s}] \\ \hline \text{water vapor} & \text{air} & 2.6 \times 10^{-5} \\ \text{napthalene} & \text{air} & 6.1 \times 10^{-6} \\ \text{sodium chloride} & \text{water} & 1.2 \times 10^{-9} \\ \text{ethanol} & \text{water} & 7 \times 10^{-10} \\ \text{helium} & \text{pyrex} & 4.5 \times 10^{-15} \\ \text{cadmium} & \text{copper} & 2.7 \times 10^{-19} \\ \text{aluminum} & \text{copper} & 1.3 \times 10^{-34} \end{array}}\)
There are some simplifications I’ve made, for example diffusivity in gases generally varies linearly with pressure, so I showed the diffusivity in air at atmospheric pressure. Diffusivity in liquids can also vary with the overall concentration itself, so I reported typical average values. Also diffusivity in solids can vary greatly with temperature, so I reported values for 298 K or room temperature. But still you can usually make the following generalizations: diffusivity values in gases (at atmospheric pressure) are around \(1 \times 10^{-5} [\text{m} ^2 / \text{s}] \), diffusivity values in liquids are around \(1 \times 10^{-9} [\text{m} ^2 / \text{s}] \), and diffusivity values in solids vary widely, but are always much smaller than those in liquids and gases.
The only remaining thing we have is to reformulate the equation in a differential form. This means we take the limit at the thickness \(L \) goes to zero, the right-hand-side of the equation becomes a derivative:
\( \huge{ N_{A} = – D_{AB} \frac{d C_A}{ d x } } \)
where now \(x \) is the coordinate in the same direction as \(L \). This is the form for one direction in a Cartesian coordinate system. The more general form that is independent of coordinate systems is written as
\( \huge{ N_{A} = – D_{AB} \nabla C_A } \)
where \(\nabla \) is the gradient operator. This is the form we’ll start with when we actually solve the problem of the bubble collapse, because the bubble is spherical so we’ll use spherical coordinates.